Algebraic thinking concept map

Teresa Maguire and Alex Neill
 

 Introduction

Equality

 

 Algebraic thinking 

  

 

Commutativity and Associativity

Exploring number properties

 

Additive identity

 
Component: 
Introduction

Algebraic thinking is about generalising arithmetic operations and operating on unknown quantities. It involves recognising and analysing patterns and developing generalisations about these patterns. In algebra, symbols can be used to represent generalisations.  For example, a + 0 = a is a symbolic representation for the idea that when zero is added to any number it stays the same. 

Studying and representing relationships is also an important part of algebra.  "The language of arithmetic focuses on answers while the language of algebra focuses on relationships."1

"Algebra can be considered a generalisation of arithmetic, and the properties of mathematics allow us to stand back from the calculation and look at the generalisations." 2

Students can more easily understand algebra when they have a good knowledge of the general properties of numbers (e.g., additive identity, commutativity), the relationships among numbers, and the effect that basic operations have on numbers rather than just having a focus on finding an answer.  Many of these concepts are best taught at a young age because misconceptions which develop early on can inhibit a student's ability to work with symbols and generalisations at a later time.  By learning about number properties, students can learn to generalise about principles of mathematics.  Students can also come to have a common understanding about what they are doing when they perform calculations.

The sections in this concept map introduce some of these important ideas, and provide links to related assessment resources.  The resources are designed to provide diagnostic and formative assessment information to help elicit student understanding.  This concept map has been developed as a result of a review of the literature and research carried out with Year 4 and 5 students.

1 MacGregor, M & Stacey, K. (1999) A flying start to algebra. Teaching Children Mathematics, 6/2, 78-86.  Retrieved 17 May 2005 from http://staff.edfac.unimelb.edu.au/~Kayecs/publications/1999/MacGregorStacey-AFlying.pdf.

2 Tent, Margaret W. (2006) Understanding the Properties of Arithmetic: A Prerequisite for Success in Algebra.  Mathematics Teaching in the Middle School, 12 (1), 22 – 25.

 

Resources

Equality

The concept of equality forms an important foundation of algebraic understanding.  Students often have a purely arithmetical view of the meaning of the equals sign.  The equals sign is commonly misunderstood as a command to take an action rather than a representation of a relationship.  Therefore, when a student sees an equals sign in an equation, they want to carry out the operation that precedes it.  To them, the equals sign means "...and the answer is".   

Algebraic understanding of equality
To move towards a more algebraic understanding of equality, students need to learn that the equals sign represents quantitative sameness – in other words, the expression on the left-hand side of the equals sign represents the same quantity as the expression on the right-hand side of the equals sign.  The equals sign means "is the same as".   Without this understanding students will find it very difficult in the future to work with equations where, for example, there are unknowns on both sides of the equals sign,  e.g., 2x + 5 = x + 10.

Number sentences
To encourage students to develop a more algebraic view of equality, they can be challenged by having alternative forms of number sentences presented to them, such as 9 = 3 + 6 or 2 + 7 = 5 + 4.  Students who are used to seeing number sentences in the form a + b = c do not see 9 = 3 + 6 as a "proper number sentence".  They claim that it is backwards and try to turn it around so the equals sign comes at the end or swap the = and + signs and state that it is false because 9 + 3 does not equal 6.  These alternate forms of number sentences can be introduced to students from a very young age.

Cuisenaire rods (or Animal Strips)
Another activity that can be started with young students is writing equivalent statements for numbers.  Use Cuisenaire rods or Animal Strips to create these and encourage students to put the equals sign at the beginning of the number sentence.  

Can be written as 10 = 4 +3 +3.
   
Snakes (9) can be written as 9 = 4 + 3 +2.

Examples of number sentences generated by a class of Year 4 students using Cuisenaire rods are listed below:

10 = 4 + 3 + 3
9 = 4 + 4 + 1
2 + 4 + 2 = 8		 10 = 1 + 2 + 3 + 4
5 + 2 + 2 = 9
8 = 3 + 1 + 1 + 1 + 1 + 1		 1 + 3 + 6 = 10
9 = 3 + 3 + 3
7 + 1 = 8

An alternative to this activity is to say to students "My number is 12, how many different ways can you write it?"

Balance
The concept of balance can be used to reinforce the idea of equality – both sides of the number sentence need to be the same, the equation needs to balance.  A set of simple balance scales with different coloured blocks (or other small items all of the same weight) in each pan can be used as a visual stimulus. Commercially available number equaliser balances can also be used to explore this concept.

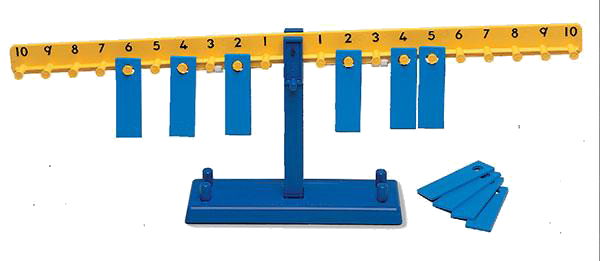balance-pans.png number-equaliser-balance-scales.png

Some things to avoid
The equals sign is sometimes used in contexts other than in a mathematical equation e.g., MATHS = FUN or John = 9.  These statements may lead to a misinterpretation or misunderstanding of the equals sign. Another common misuse of the equals sign is when, for problems such as 3 + 5 × 2, equality strings are written down e.g., 3 + 5 = 8 × 2 = 16, which implies that 3 + 5 = 16.  Encourage students to rewrite their steps underneath each other, i.e.,    

               3 + 5 = 8
               8 × 2 = 16

or develop their own symbol or sign to use, for example an arrow:

3 + 5 = 8 8 × 2 = 16.

Calculators can further confuse student understanding of the equals sign because when the key on a calculator is pressed, the answer is given.  This can reinforce the idea that equals is an operation meaning "and the answer is".

Further reading
This article summarises some of the issues surrounding the understanding of equality: Darr, C., (2003). "The meaning of equals."  Set: Research information for teachers. 2, 4-7. 

Closure
Students who give the answer '10' to a problem such as 4 + 6  =  +  5 are often looking for 'closure'.

Closure is a concept defined by Kevin Collis2 (1975a, 1975b).  In his development of levels of closure, he identified the lowest level as when a student feels that a problem requires a unique result – a single number that replaces two other numbers connected by an operation.  This is typically the way students work out answers in arithmetical problems, such as 4 + 5 = x.  In this problem x = 9.  If students are only exposed to problems of the type a + b = c, when they encounter a problem such as 4 + 6 =  + 5, they instinctively want to find the unique solution to 4 + 6, rather than explore the relationship between the two equations.  Alternatively, some students might give the answer '15' to this problem – they find a unique solution by adding all available numbers.

Collis goes on to define three more levels of closure.  At the second level students can work with combined elements without actually replacing the elements with a unique answer (e.g., they know that 7 + 8 > 1 + 2 without having to solve each side of the equation).  They can also use two operations (e.g., 7 + 6 - 8). 

At the third level, students can work with formulae such as Volume = L × W × H as long as each letter stands for a unique number and can be "closed" at any stage.  In other words, students are able to substitute values into a formula to get a result, thus if L = 5, W = 3 and H = 4, the Volume is 60 cubic units.

The fourth and final level occurs when a student can deal not only with variables in a formula, but is able to discuss what the effect of changing one variable would have on other variables in the formula without having to substitute or work with actual numbers.  For example, if the Length (L) in the Volume formula is doubled, then the Volume will also double.  Students can also see that in a problem such as 4 + = 3 + 7, if the 3 is replaced by a larger number, the number in the box would need to be larger also.

Young students have difficulty with what Collis3 (1974, cited in Herscovics & Linchevski, 1994) called "Acceptance of the Lack of Closure" (ALC).  This idea becomes important when working with algebraic expressions or formulae (beginning at around Level 4 of the curriculum) where a solution may be in the form x + 4 or 8 × a, and cannot be "closed".  This difficulty can also lead to students giving the answer 7x to the problem 3x + 4 = because they feel the need to find a unique solution.

2 Collis, K. (1975a). The Development of Formal Reasoning, Report of a Social Science Research Council sponsored project (HR2434/1)     carried out at the University of Nottingham School of Education.  New South Wales, Australia: University of Newcastle.

  Collis, K. (1975b). A Study of Concrete and Formal Operations in School Mathematics: A Piagetian Viewpoint.  Victoria, Australia: Australian     Council for Educational Research.

3 Herscovics, N., & Linchevski, L. (1994) A Cognitive Gap Between Arithmetic and Algebra.  Educational Studies in Mathematics, 27, 59 – 78.

Resources

Additive identity

The additive identity property seems to be intuitively understood by students, even very young ones.  The idea that adding or subtracting zero leaves the original number intact is relatively easily grasped 5. However, because of its later application in solving algebraic equations, this number property is an important one to explore.  It is also important for students to be able to articulate their understanding of number properties. The additive identity property can be expressed in four different ways, and each way can be written in two equivalent forms:

5 + 0 = 5 or  5 = 5 + 0   (a + 0 = a  or  a = a + 0)
0 + 5 = 5 or 5 = 0 + 5   (0 + a = a  or  a = 0 + a)
5 – 0 = 5 or 5 = 5 – 0   (a – 0 = a  or  a = a – 0)
5 – 5 = 0 or 0 = 5 – 5   (a – a = 0  or  0 = a – a)

It is useful for students to see each rule written in its alternative form to help consolidate the idea that the equals sign does not have to be the penultimate symbol in an equation, but can also be near the beginning. 

5 The 2005 NEMP report (http://nemp.otago.ac.nz/ - Mathematics/Number & Algebra/Maths Helper) showed that just over 50% of Year 4s and just under 75% of Year 8s were able to identify 0 as the number that you add to or take from 8 to keep it the same.

Developing conjectures
The resources Looking at zero and Number sentences can be used as diagnostic tools to identify any common misunderstandings students might have about zero.  They can also be used as discussion starters so that a group or class can develop statements or conjectures about what happens when zero is added to, or subtracted from, a number.  Students often try out a range of different numbers in order to test their ideas.  It is important to encourage students to consider whether their conjecture (theory) works for all numbers.  In this way, students are beginning to generalise about number properties in an algebraic way. The rules or conjectures developed by a class can be displayed and/or the student who came up with the concept could have their name attached to it, e.g., "Jonathan's Rule". Examples of conjectures developed by Year 4 students:

  • "When you add zero with a number it doesn't change the number that you started with." (a + 0 = a)
  • "When you subtract zero from a number it doesn't change the number you started with." (a – 0 = a)
  • "If you take the number you started with away from the number you get 0." (a – a = 0)

Writing number sentences
To consolidate understanding, encourage students to create their own number sentences which make use of the additive identity.  These could be in the form of True/False or open number sentences.  Examples generated by students can be used to formatively assess student understanding of the additive identity.    Examples of True/False number sentences from Year 4 students include:

  • 2 + 8 = 10 + 0 (T)
  • 78 + 0 = 87 (F)
  • 4 000 + 0 = 400 (F)
  • 678 + 9 = 678 + 9 + 0 (T)
  • 100 000 + 0 + 2 = 100 002 (T)
  • 500 – 0 = 499 (F)
  • 1000 + 0 = 999 + 1 (T) 

Some examples of open number sentences, also from Year 4 students include:

  • 11 – 46 + 46 =
  • 999 – 0 + 99 – 999 + 0 =
  • 100 250 + 100 000 – 100 250 =
  • 225 – 25 + 200 + 25 – 200 =

Applying the property
Once a common understanding of the additive identity is established, application of the property can then be explored.  Resources Solving problems, Solving simple equations, and Solving equations are designed to encourage students to "find the zero" embedded in an equation.  For example, in the problem 34 + 62 – 34 = , students "find the zero" by subtracting 34 from 34 and find the answer, 62, without any calculation.  This pre-algebraic skill is important because when students begin solving algebraic equations such as 2x – 5 = x + 3 they will need to understand why "change the side, change the sign" works and that it actually involves subtracting a number or unknown quantity (x) from itself to make 0.  This can be seen in the way this problem is solved in the steps below:

      2x – 5 = x + 3

2x – x – 5 = x – x + 3  [subtract x from both sides to 'make 0' and eliminate an x from one side of the equation]

        x – 5 = 0 + 3

  x – 5 + 5 = 3 + 5       [add 5 to both sides to 'make 0' and leave x on its own on one side of the equation and a number on the other side]

        x – 0 =  8

              x = 8

After finding the value of x, replace x in the original equation with this value to check for accuracy.  If x = 8, then 2x - 5 = x + 3 will be: (2 x 8) - 5 = 8 + 3, 16 - 5 = 11, 11=11.

 

Resources

Commutativity and Associativity

Two properties that are frequently used to solve problems are the commutative and associative properties of addition.  As with the additive identity, students often have an intuitive understanding of these properties and use them without necessarily realising there are underlying mathematical principles being applied.

The commutative property states that a + b = b + a. In other words, the order of the numbers can be changed without changing the value of the expression. The associative property states that (a + b) + c = a + (b + c). In other words, regrouping the numbers when adding does not change the solution.

These two properties are commonly applied in situations where students use numeracy strategies, such as counting on, to solve a problem.  For example, if students are asked to solve 3 + 14, they are more likely to count on from 14 to get to 17, i.e., the problem becomes 14 + 3.  They naturally apply the commutative property without realising that is what they are doing.

In another example, students might use firstly the commutative property, then the associative property to solve the following problem: 28 + 17 + 12 = ....  In this case students would be encouraged to put compatible numbers together to make a tidy number which would then be easier to add. The next step might look like this: 28 + 17 + 12 = 28 + 12 + 17

The commutative property has been used here – it does not matter which order the 28 and 17 are in, their position in the problem will not affect the result.  In her article on number properties, Margaret Tent (2006) 6 talks about 'moving wagons' to describe the commutative property.  Every time two numbers are switched around in a problem it is like they have been put on wagons, or trolleys, and moved from one place to another.   The diagram below shows a way to represent this movement:

The next step to solve the problem would be to regroup the numbers.  To ensure the compatible numbers are dealt with first, they are bracketed.

28 + 17 + 12 =  28 + 12 + 17

                      =  (28 + 12) + 17

                      =  40 + 17

                      =  57

Here the associative property has been used.  Margaret Tent uses the analogy of 'hand-holding' to describe what is happening to the numbers.  Those that are in the brackets are added first – they are 'holding hands' and 'excluding' the other number – 17 in this case.

In another example where a numeracy strategy is applied, students solving the basic fact problem 8 + 5 might apply the associativity rule thus: 

8 + 5 = 8 + (2 + 3)

         = (8 + 2) + 3

         = 13

While students solve these types of problems regularly, the underlying number properties being used are not often articulated or made explicit.  Giving names or labels to these processes empowers students and enables them to explain more clearly what they are doing.  With a common language for explaining processes, a group or class of students is better able to understand how each person is solving problems.  The ability to articulate mathematical processes and strategies is a crucial part of the development of confident and capable mathematicians. Through the use of True/False number sentences or open number sentences, these concepts can be explored in the same way as the additive identity property. Number sentences that can be identified as True or False, such as:

                                            3 + 8 = 8 + 3

                                       31 + 42 = 42 + 13

                                       25 + 46 = 46 + 25

                                  165 + 785 = 785 + 868

can be used to generate discussion and develop a conjecture or rule about the commutative property.  Students can also be encouraged to write their own True/False number sentences. Here are some examples of student generated rules about the commutative property.  The first comes from a class of Year 4 students, while the second comes from Carpenter, Franke & Levi's book and is written in the natural language of children.

  • “It doesn’t matter if the numbers are swapped around on each side of the number sentence.  If the numbers are the same, the number sentence will still balance.”
  • ‘When you add two numbers, you can change the order of the numbers you add, and you will still get the same number.” 7

The concept of equality, meaning quantitative sameness, needs to be firmly in place prior to students exploring number properties.  In the first conjecture above the student refers to the idea of "balance" to explain commutativity.  Without this prior understanding, the concept of commutativity would be more difficult to describe. To reinforce this idea, balance pans (as used in resource Balance pans) can be used to model commutativity. A further way to model commutativity is through the use of number lines.  Students can be presented with a number line such as: 

and asked what number sentence it represents (6 + 2 = 2 + 6).  They can then be given number sentences e.g. 11 + 3 = 3 + 11 or 14 + 7 = 7 + 14 and asked to show these on blank number lines.

6 Tent, Margaret W. (2006) Understanding the Properties of Arithmetic: A Prerequisite for Success in Algebra.  Mathematics Teaching in the Middle School, 12 (1), 22 – 25.

7 Carpenter, T., Franke M. L., & Levi, L. (2003). Thinking Mathematically. Integrating Arithmetic and Algebra in Elementary School.  Portsmouth ,  NH : Heinemann, p. 55.

A common misconception
Students can often incorrectly over-generalise the commutative property to subtraction, i.e. they believe that 7 – 5 = 5 – 7 8 and will even read the problem 5 – 7 as "seven minus five".  Have them try out the problem using manipulatives to see if 7 – 5 is the same as 5 – 7.  Alternatively, getting students to make up their own story problems to describe the number sentences can help start a discussion about whether the two expressions are equal. 9

8 In the 2005 National Education Monitoring Project (NEMP) report (http://nemp.otago.ac.nz/  -  Mathematics/Number & algebra/Maths Helper) 40% of Year 4s and 20% of Year 8s stated that 4 – 2 and 2 – 4 were the same.
9 Vance, J. H., (1998) Number Operations from an Algebraic Perspective.  Teaching Children Mathematics, 4, 282-285 (http://www.learner.org/channel/courses/learningmath/algebra/pdfs/AlgPerspective.pdf)

Further reading
Glenda Anthony and Margaret Walshaw explore some of the ideas students have about commutativity in the following article:
Anthony, G., and Walshaw, M., (2002) Children's notions of commutativity: Do we need more than "turn-arounds"?  Set: Research information for teachers. 3, 1-4.