Multiplication boxes and triangles II

Multiplication boxes and triangles II

Pencil and paper
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about finding numbers to complete a multiplication number sentence.
Look at the multiplication number sentence:
  × × 2      =    48  
 
In the table below, list all the possible combinations of numbers that can make the number sentence true. 
You can use any numbers to complete the number sentence.
 
× × 2      =     48
 
 
black triangle black box
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
Task administration: 
This task is completed with pencil and paper only.
Level:
3
Curriculum info: 
Maths, Number and Algebra, Equations and expressions
Key Competencies: 
Using language, symbols, and texts
Keywords: 
combinations, multiplication
Description of task: 
Students explore multiplicative relationships by completing multiplication equations.
Learning Progression Frameworks
This resource can provide evidence of learning associated with
Multiplicative thinking, sets 4-5
Using symbols and expressions to think mathematically, set 4
within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.
Answers/responses: 
  Y6 (11/2006)

1 & 24
2 & 12
3 & 8
4 & 6
6 & 4
8 & 3
12 & 2
24 & 1

2 marks (for 4 or more correct combinations)
or
1 mark (for 1 - 3 correct combinations)

 

Accept other pairs of numbers that multiply to 24*.

difficult
 
moderate
Based on a representative sample of 163 students 

* Most students responded using whole numbers only.  A few, however, gave answers which used decimal numbers e.g. 1.5 × 16.

Teaching and learning: 
This resource is about exploring relationships between numbers.  In this case, it is the relationship between the given number and the total that goes in the square and triangle.  The question underlying the problems is: What should the numbers that go in the triangle and the square equal in order to make the number sentence true?
Diagnostic and formative information: 
Some students gave a list of combinations that added to 24. Others gave lists of numbers that added or multiplied to 48 (ignoring the given × 2).
Next steps: 
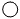If students are struggling to see the relationships between the numbers and are unable to come up with combinations, cover up the triangle and square with your fingers (which is equivalent to the problem  × 3 = 18) and ask them, "What number needs to go under my fingers to make the number sentence true?"  Remove your fingers and look for combinations that will multiply to that number.  For example in part a) i) cover up the square and triangle and ask, "What number needs to go under my fingers to make the number sentence true?" The answer is 6.  Lift your fingers and ask students what numbers could go in the square and triangle that multiply to 6.
If students are having difficulties with the idea of combinations, use a simple concrete example such as: There are two cages joined by a tunnel. Seven pet mice are in the cages.  List all the possible ways that the 7 mice could be in the two cages.  Explore whether students have found all the possible combinations.  Increase the difficulty level of the problem by adding extra numbers or making the problem multiplicative rather than additive.

If students have given an additive rather than a multiplicative response, have them look carefully at the operations in the number sentence.

If students have given a list of combinations that adds or multiplies to a number other than 24, encourage them to work out what the numbers in the square and triangle should multiply to and have them find those possible combinations.

Students who listed some or all of the possible combinations that totalled 24, could be asked, "Have you got all the possible combinations?" and then "How do you know you have all the possible combinations?"

Exploration of the number property commutativity, can be initiated using this resource: have students share the combinations they found.  This should illuminate the commutative nature of finding all the possible combinations and promote discussion over whether, for example,  12 × 2 is the same as 2 × 12.

If students are comfortable exploring combinations using triangles and squares, these types of problems could be a way of introducing them to pronumerals, by replacing the triangle and square with x and y.
Other resources

•  For more information about algebraic thinking and commutativity refer to the Algebraic Thinking Concept Map and go to the section on Commutativity and associativity.
•  For further information about using discourse in the classroom refer to Assessment Strategies: Mathematical Classroom Discourse.