Working out the unknown number
Show how to find the unknown number (represented by a letter) in each of the following equations.
a) 544 + x – 544 = 238 – 238 + 112
|
|
|
b) 256 + r = 19 + 172 + 256 – 172
|
|
|
c) 358 + 13 – 13 + m = 179 + 358 – 179
|
|
|
d) y + 55 = 678 + 54
|
|
|
e) 88 + 47 = 86 + 46 + t
|
|
|
f) 65 – q = 66 – 18
|
| Y8 (11/2007) | ||
| a) |
112 |
difficult |
| b) |
19 Uses a complete and workable strategy. |
difficult very difficult |
| c) |
0 Uses a complete and workable strategy. |
difficult very difficult |
| d) |
677 Uses a complete and workable strategy. |
very difficult very difficult |
| e) |
3 |
difficult difficult |
| f) |
17 Uses a complete and workable strategy. |
difficult very difficult |
NOTE: A 'complete and workable strategy' includes applying number properties and relational thinking or using accurate calculations to solve the equation. Students may have described or used a 'complete and workable strategy' but not got the correct answer.
While students found this task difficult to accurately complete, it does provide opportunities to investigate number properties and relational thinking.
Questions a), b) and c) exploit the additive identity/additive inverse and commutative number properties; while questions d), e), and f) can be solved by using relational thinking.
| Common error | Likely calculation | Likely misconception | |
|
a)
b)
d) f)
|
0 1088 238 275 358 623 |
544 + 0 = 544 544 + 544 = 1088 544 + 238 – 544 = 238 275 = 19 + 172 + 256 – 172 358 + 13 – 13 = 358 or 358 = 179 + 358 – 179 623 + 55 = 678 678 + 54 = 732 86 + 46 = 132 88 + 47 = 135 65 – -1 = 66 65 + 1 = 66 66 – 18 = 48 |
Performs calculations on one side of the equation only, sometimes ignoring or changing operators. |
|
a)
b) |
306 782 – 237/237 191 |
544 – 238 = 306 544 + 238 = 782 256 – 237 = 19 191 = 19 + 172 |
Works only with the numbers surrounding the equals sign, sometimes ignoring or changing operators. |
| c) | 179 |
358 + 13 – 13 + 179 = 179 + 358 -> 358 + 179 = 179 + 358 |
Ignores the final – 179 and applies the commutative number property to the remaining number sentence. |
|
d) e) f) |
679 1 19 |
678 + 1 = 679 47 – 46 = 1 18 + 1 = 19 |
Attempts to use relational thinking but adds instead of subtracts or only applies it to one pair of related numbers. |
If students are ignoring or changing operators and performing calculations on the numbers immediately surrounding the equals sign or on one side of the equation only, it is likely that they have a misconception about the concept of equality. They believe that the equals sign means "and the answer is" rather than representing quantitative sameness, i.e., the quantity on the left-hand side of the equals sign needs to be the same as the quantity on the right-hand side of the equals sign. The Level 3 resources Equal number sentences II and Equality explore the concept of equality through True/False number sentences and open number sentences. For further information and resources on the concept of equality, refer to the Algebraic Thinking Concept Map: Equality.
Calculating to solve the equations - not recognising the additive identity/commutative properties
In questions a) through c), if students are calculating to solve these equations, have them explore the additive identity and commutativity properties. The Level 3 resources Looking at zero and Number sentences II. Both offer opportunities to explore and discuss the additive identity property through True/False number sentences and open number sentences. Solving Equations has similar equations to this resource but uses simpler numbers.
For more detailed information about the additive identity property, click on the link, Algebraic Thinking Concept Map: Additive identity. The commutative property can be explored in a similar way using True/False number sentences. An alternative way of looking at commutativity is by using number lines. The resource Commutative number lines II provides examples of this. Further information about commutativity can be found at Algebraic Thinking Concept Map: Commutativity and Associativity.
Calculating to solve the equations without using relational thinking
If students are calculating the answers in questions d) through f), have them look carefully at the numbers on each side of the equation and see, for example, that 55 and 54 are only one away from each other. Ask students if y needs to be one bigger or one smaller than 678 to make the equation balance. Students need to have an algebraic understanding of the equals sign in order to solve this type of equation. To build up an understanding of how to solve equations using relational thinking, start with small numbers and explore the relationships there first.
For example, start with x + 5 = 10 + 6. Ask students to find a way to solve the equation that does not involve calculating the answer to one side of the equation and using that to solve for the other side. Change one of the numbers and explore again, e.g., x + 5 = 11 + 6. Ask students to work out what happens to x when the 10 changes to 11. Keep changing one of the numbers until there are enough examples to make a generalisation. Move on to bigger numbers and explore whether the generalisation or the rule works each time.
Subtraction equations
For subtraction equations, such as question f), number lines can be useful. Students need to understand that subtraction is about difference and the difference between the numbers needs to remain the same on both sides of the equation. It is also helpful for students to understand that subtraction is the inverse of addition.
Explore the ideas using small numbers and build up a general rule before giving students larger numbers to work with. For example, start by using a simple equation such as 5 – 3 = 6 – x and draw a number line showing the two sides of the equation:
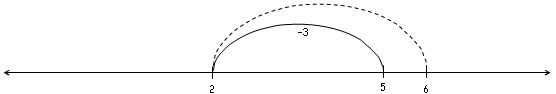
Discuss the idea that both lines need to end up at the same point and ask "What value is the dotted line?" Students can visually see what the number is that has to be subtracted. Emphasise that the difference between 5 and 3 is the same as the difference between 6 and x (6 and 4).
When using larger numbers, it is important for students to realise that they don't need to know what the answer to the given subtraction equation is. The focus is on what the difference between the given number and the unknown is. For example, given the equation 147 – 38 = g – 39, the following diagram can be used:

Note that no "answer" is given for the 147 – 38 part of the problem–the emphasis is on the relationship between g and 147; is g greater or smaller than 147?

