Grid triangles

Grid triangles

Pencil and paper
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about continuing a pattern of triangles, and finding a rule.

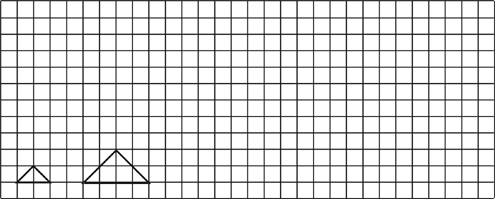
a)
On the grid of squares above, draw the next 2 shapes in this series of triangles.
 
b)
Complete this table, giving the area of each triangle:
 
Height of triangle (number of squares high)
1
2
3
4
Number of squares in the triangle (area)
1
4
 
  
 
c)
 
How many squares will be in a triangle of height ...
  
 
i)
 
ii)
 
 
10 squares? __________ squares
 
20 squares? __________ squares
 
d)

 
Explain or write a rule that describes how to work out the total number of squares (area) needed for any given height of triangle.
 
 
 
 
 
e)

 
Show how to work out the height of a triangle that has 81 squares in it.
 

 
 
 
 

Height = __________ squares
Task administration: 
This task is completed with pencil and paper, and a ruler.
Level:
4
Curriculum info: 
Maths, Number and Algebra, Patterns and relationships
Keywords: 
spatial patterns, functional rules, square numbers, quadratic equation
Description of task: 
Students draw the next two triangles in a spatial pattern, calculate the areas of a range of triangles, work out the height of a triangle given its area, and write a rule for the pattern.
Learning Progression Frameworks
This resource can provide evidence of learning associated with
Patterns and relationships, sets 5-6
within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.
Answers/responses: 

 

Y8 (11/2004)

a)

  

easy

b)

 

9, 16.
[Accept answer consistent with drawings in part a).]

moderate

c)

i)
ii)

100
400

difficult

d)

 

For any 1 of:

  • multiplies the height of the triangle by itself;
  • squares the height of the triangle (h2 = a);
  • identifies area as 1/2 bh (measurement).

moderate

e)

 

9
Uses a strategy that involves any 1 of:

  • √81 = 9 or 92 = 81 or
  • other working that shows that 9 squared is 81.

NOTE: the answer does not have to be correct to get a mark for showing a correct strategy/working.

difficult

moderate

Results are based on a trial set of 228 Y8 students.

Diagnostic and formative information: 
  Common error Likely calculation Likely misconception
c) ii) 200 2 × 100 Correctly uses 102 to solve c) i) but then doubles that answer rather than finding 202.
c) i)
    ii)		 20
40		 2 × 10 Incorrectly identifies the relationship between area and height (possibly only focussing on the relationship between height and base (b = 2h) - not the area).
NOTE: most other incorrect responses for ii) were 2 × i).
e) 40.5 81 ÷ 2 Area is half the height (possibly confusing the fact that the height is half the length of the base).
e) 6561 81 × 81 Reversing the rule, i.e., finding the square rather than the square root.