Square flower beds

Square flower beds

Pencil and paper
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about estimating the square root of an area.

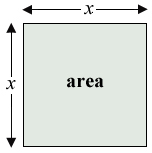
Liam, Beth and Caleb each have a square flower-bed. The length of each side of the square flower-bed (x) is equal to the square root of the area of the flower-bed.

a)
Liam has a square flower-bed with an area of 25 m2. What is the length of each side of his flower-bed?
 
__________ m
  
b)
Beth has a square flower-bed with an area of 10 m2. The length of each side is somewhere between 3 and 4 metres.
Without using a calculator explain how you know the length is within this range.
 
 
 
 
 
 
 
c)
Caleb has a square flower-bed with an area of 40 m2.
 
 
i)
 
ii)
The length of each side is between ___________ and __________ metres.
 
Without using a calculator explain how you know the length is within this range.
 
 
 
 
 
 
Task administration: 
This task is completed with pencil and paper only.
Level:
5
Curriculum info: 
Maths, Number and Algebra, Number Strategies
Key Competencies: 
Using language, symbols, and texts
Keywords: 
square roots, estimation
Description of task: 
Students calculate an exact square root and estimate approximate square roots by finding the length of the square flower bed of a given area, and give an explanation for their answers.
Curriculum Links: 
Key competencies
This resource involves explaining why estimates for sqaure roots must lie within given ranges. This relates to the Key Competency: Using language, symbols and text.

For more information see http://nzcurriculum.tki.org.nz/Key-competencies.

Learning Progression Frameworks
This resource can provide evidence of learning associated with
Multiplicative thinking, sets 8-9
within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.
Answers/responses: 
   

Y10 (11/2000)

a)

5

easy

b)

Any 1 of: 

  • 32 = 9 and 42 = 16
  • 32 is too low, and 42 is too high
  • √ 9 = 3 and √ 16 = 4

NOTE: do not accept "because √ 10 is between 3 and 4".

difficult

c)

i)
ii)

6, 7
Any 1 of: 

  • 62 = 36 and 72 = 49
  • 62 is too low, and 72 is too high
  • √ 36 = 6 and √ 49 = 7

NOTE: do not accept "because √ 40 is between 6 and 7".

moderate
difficult
Diagnostic and formative information: 
  Common error Likely calculation Likely reason
b)
c)		 Uses a calculator.
Uses a calculator.		    
a)
c)		 6.25
9, 11		 25 ÷ 4
40 ÷ 4 = 10		 Confuses area and perimeter.
For more information about computational estimation see the Computational estimation concept map.