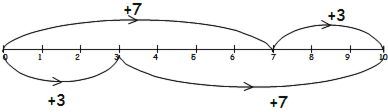
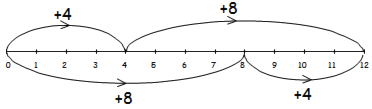
Commutative number lines
This task is about using number lines to show number sentences.
| a) |
Write the number sentence that each number line shows in the boxes below.
|
||||||
|
i)
|
|
||||||
|
ii)
|
|
||||||
| b) |
Use the number lines below to draw in each number sentence.
|
||||||
|
i)
|
|
||||||
|
ii)
|
|
||||||



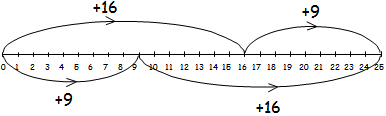
 + 29 = 81 explores commutativity when finding an unknown. (Book 5: Teaching addition, subtraction, and place value (pdf)).
+ 29 = 81 explores commutativity when finding an unknown. (Book 5: Teaching addition, subtraction, and place value (pdf)).