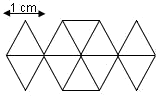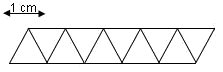Area and perimeter of four shapes

Area and perimeter of four shapes

Auto-markingPencil and paperOnline interactive
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about finding the shape with the largest area and longest perimeter.
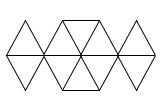
Shapes A to D are made up of identical equilateral triangles.
The three sides of each triangle are each 1 cm long.
 
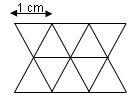
shape A made using triangles
Shape A
 
shape B made using triangles
Shape B
 
shape C made using triangles
Shape C
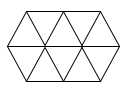
shape D made using triangles
Shape D
 

Question

a)  Which of the shapes has the largest area?

    • shapeA.png

    • shapeB.png

    • shapeC.png

    • shapeD.png

    • All the shapes have the same area

Question

b)  Which of the shapes has the longest perimeter?

    • shapeA.png

    • shapeB.png

    • shapeC.png

    • shapeD.png

    • All the shapes have the same perimeter

Task administration: 
This task can be completed online and has auto marking displayed to students.
Level:
4
Curriculum info: 
Maths, Geometry and Measurement, Measurement
Key Competencies: 
Thinking
Keywords: 
area, perimeter
Description of task: 
Students identify which of four shapes created from equilateral triangles has the largest area and which has the largest perimeter.
Curriculum Links: 
This resource can help to identify students' understanding of area and perimeter.
Learning Progression Frameworks
This resource can provide evidence of learning associated with
Measurement sense, sets 6-7
within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.
Answers/responses: 
   

Y8 (06/2000)

a)

All the shapes have the same area

easy

b)

Area-perimeter-shapeB.png

moderate
 
Diagnostic and formative information: 
 

Common error

Likely reason

b)

35% of students selected option

Area-perimeter-shapeC.png

Confused longest perimeter with longest shape.
Next steps: 
Extension  

  1. Draw shapes with the ten equilateral triangles with as many possible perimeters as you can. The triangles must either touch just at a vertex or have one side wholly in common. (All even numbers between 8 and 30 have solutions)
  2. What is the maximum possible perimeter? (Answer: 30 cm).