Continue the patterns II
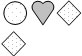
a) i) Draw the next three shapes in this pattern.

ii) Draw the 15th shape in this pattern.
iii) Show or explain how you worked out what the 15th shape was.
a) i) Draw the next three shapes in this pattern.

ii) Draw the 28th shape in this pattern.
iii) Show or explain how you worked out what the 28th shape was.
| Y6 (06/2009) | |||
| a) |
i) ii) iii)
|
 An explanation about how they worked out part ii):
|
very easy moderate easy
|
| b) |
i)
ii)
|
 An explanation about how they worked out part ii):
|
very easy moderate easy
|
These questions are about identifying the type of shape and type of shading of a pattern and being able to work out elements of the pattern for a given ordinal position. What underlies these attributes is whether students can identify the "repeating unit" of the pattern.
Diagnostic and formative information
Almost 90% of Year 6 students correctly continued the repeating pattern in part i) of questions a) and b). However, asking them to identify the shape at a given ordinal position (part ii) in the pattern was more difficult. This proved to be a good indicator of their understanding of the pattern, and their ability to project the pattern beyond the drawings provided.
Correct strategies (not necessarily correct answers)
Across all the three questions the most common strategy for answering part ii) was counting on from the shapes shown, followed closely by continuing the pattern by drawing the shapes, and other strategies that involved either skip counting to the ordinal position or building up to the ordinal position using the repeated unit. Interestingly, the counting strategies were not very successful for either working out more complex shape patterns, or identifying the shape for a given ordinal position.
Drawing the remaining shapes to work out the shape for a given ordinal position
Many of the students continued to draw the patterns to answer the question in part ii. Students simply continued the pattern until they reached the ordinal position required and identified the shape. The proportion of students who used this strategy was a third for question a), and a quarter for question b). This may relate to the increasing difficulty of the questions.
Counting on from the shapes
Another strategy students used to find the shape of a given ordinal position was counting on from the pattern without using the basic repeating pattern but the shapes shown and counting on from them. If students take into account how the patterns continues (that it has a repeating unit) this strategy, although time consuming, could be accurate and less prone to error than drawing all the shapes. The proportion of students who used this strategy was approximately one third for both patterns.
Recognising the basic repeating unit
Identifying the repeating unit and counting up, e.g., for question b), students could identify that the basic repeating unit was the first six shapes  and that they could work out other shapes by simply counting these first 6 shapes and counting over them again:
and that they could work out other shapes by simply counting these first 6 shapes and counting over them again:
This strategy could also be supplemented by students stating that every 6th shape is  , skip counting to 24th and then adjusting to get the 28th shape. This is effectively like skip counting and compensating.
, skip counting to 24th and then adjusting to get the 28th shape. This is effectively like skip counting and compensating.
Misconceptions
Not recognising what is being repeated and restarting the pattern at the end of the line. One misconception that underlay many student errors with repeated patterns was that the repeated unit was not recognised.
Part i): Some students indicated that the pattern either repeats the shapes that have been shown already, or the shapes that are shown after drawing, and then starts from the beginning (the first shape) again. For example, in questions a) and b) students would have drawn AAA or BBB orrespectively - very few students did this, possibly because the patterns were not sufficiently difficult to challenge their current counting strategies for solving.
Part ii): Asking for the shape of a given ordinal position in the pattern checks whether students' strategies are accurate. Students who got part i) correct and then identified an incorrect shape for part ii) may not fully understand how the pattern really worked (repeated). Although they did not start the shapes again for part i), about a fifth of students (across both questions) incorrectly answered  and
and  (for questions a and b respectively) which suggests they reverted to starting the pattern again when they reach the end of the line, rather than continuing the pattern (indefinitely)
(for questions a and b respectively) which suggests they reverted to starting the pattern again when they reach the end of the line, rather than continuing the pattern (indefinitely)
All three patterns have two attributes that are changing through the pattern - the type of shape and the type of shading. As the questions progress the patterns become more complex as there are more variations of each attribute, and the repeating unit more difficult to identify.
Does not continue the pattern
Students who could not continue the repeating pattern may need more practice continuing and describing more simple repeating patterns (Shape patterns, Missing shapes, and Bag of shapes) and working with beads: Necklace patterns and Repeating bead patterns to see how repeating patterns can be built up. They may also need to explore different shapes using attribute blocks, or assessment resources exploring shapes.
Not able to identify the correct shape for a given ordinal position
Students who could not identify the shape for a given ordinal position may need practice identifying and describing what is happening with the type of shape and type of shading in simple repeating patterns. For example, for the pattern in question b) every 3rd shape is a diamond (from three shapes); and every second shape is either dot or grey patterned.
Therefore there are 3 types of shape and 2 types of shade (6 different shapes in the pattern). 
They can be asked "When does the pattern start to repeat?" to help them identify the basic repeating unit. Ask them to mark it out. Students would also need to be aware of the convention of the pattern continuing forward and backward indefinitely (see below for practical ARB resources involving repeating patterns).
Students who correctly used counting strategies
Get students who used drawing strategies or counting strategies to work out the nth shape in the sequence. Ask "Can you find a quicker or easier way to work that out?" Students could also be asked how they might use a non-counting strategy for working out these questions. Discuss all the different strategies that other students use to work out the nth pattern and explore which one(s) are the most efficient. Also simply asking them to find the basic repeating unit can support a more efficient strategy (see above).
For more information about algebraic patterns, see the Algebraic Patterns Concept Map.


 )
) )
) ).
). ) 6th, 12th, 18th, 24th & 4 more to 28th or 6th, 12th, 18th, 24th, 30th & 2 less.
) 6th, 12th, 18th, 24th & 4 more to 28th or 6th, 12th, 18th, 24th, 30th & 2 less. )
) ).
).