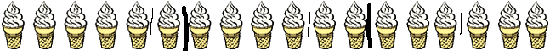Equal sharing

Equal sharing

Pencil and paper
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about sharing out equal amounts.
a)

 
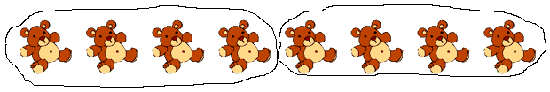
Show how to share these 8 teddy bears between 2 people, so that both people get the same number of teddy bears.

 
 

How many teddy bears does each person get? _____
 
b)

 
Show how to share 12 Christmas trees amongst 4 people, so that each person gets the same number of Christmas trees.

 
How many Christmas trees does each person get?_____
c)
Show how to share 18 ice creams trees equally between 3 families.
 
 
 
   
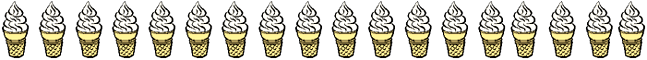How many ice creams does each family get? _____
Task administration: 
This task is completed with pencil and paper only.
Level:
1
Curriculum info: 
Maths, Number and Algebra, Number Strategies
Keywords: 
partitioning, equal sharing, division
Description of task: 
Students show how to break given amounts into a number of equal sized groups and find the amount each person gets.
Curriculum Links: 

This resource can help to identify students' ability to use simple grouping strategies to solve partitive division problems.

Learning Progression Frameworks
This resource can provide evidence of learning associated with
Multiplicative thinking, sets 1-2
within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.
Answers/responses: 

a)

4 and
appropriate strategy shown, e.g.,

     1                  2               3                4


or 2 × 4 = 8 or 8 ÷ 2 = 4

difficult

b)

3 and
appropriate strategy shown, e.g.,
  1          2          3         4         1          2          3         4        1          2          3         4

(count up the 1's, 2's 3's and 4's and that is how many each person gets)
or 4 × 3 = 12 or 12 ÷ 4 = 3

difficult

c)

6 and
appropriate strategy shown, e.g., trial and improvement (dotted lines indicate initial attempt).

 1       2      3     4       5      6 

or 3 × 6 = 18 or 18 ÷ 3 = 6

difficult

These results are based on a trial set of 149 Y5 students in May 2005.

Diagnostic and formative information: 
  Common error Likely calculation Likely misconception
a)
b)
c)		 4
3
6		 8 : 2
12 : 4
18 : 6		 Students performed the correct calculation but did not show how they had shared out the items.

The results of the trial indicate that while most students were able to calculate the correct answer, many had difficulty showing how they had solved the problem. This could be because they:

  • are not used to being asked to show their working, or
  • have difficulty putting their method on paper, or
  • believe that the purpose of maths is to find the right answer not to show how they got there.
Next steps: 

Students who show only the solution may not be aware of the importance of showing the processes they go through to find those solutions.  Encourage students to record all their workings. 

The strategy students use to solve the problem can indicate their stage of numeracy development.

A basic progression of increasing sophistication of equal sharing could be:

  • using materials (stage two of The Number Framework);
  • trial and error using the pictures on the page;
  • imaging the picture in their head (not needing to write down);
  • skip counting;
  • using repeating addition;
  • multiplication / division.

Students should be encouraged to develop beyond counting strategies to part-whole strategies (additive and, ultimately, multiplicative).

If students are recognising the multiplicative relationship between the total and the number of groups, and are using reverse multiplication (e.g., 6 × 3 = 18) or division (18 ÷ 6 = 3) to solve, then they could try solving similar problems with more complex numbers.

Also, the question could be asked in the form (find the number of groups):
"If there are 27 dogs and each family is getting 3 dogs, how many families are there?"
This is not as easily recognisable as a division problem.