Athletic track

Athletic track

Pencil and paper
Overview
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about circumference and perimeter.

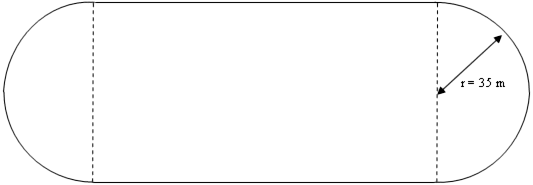
The grounds keeper has just painted a line for the inside of the Marston Athletic Track.

The line he painted is shown in the diagram below. The two end sections are semi-circles with a radius of 35 metres (m).



 

a) Show how to work out the total length of the two curved sections of the line.
 
Show your working here.

 
 
 
 
 
 
 
 
__________ m (to 1 d.p.)

 

b) The length of the line is 400 metres.  Show how to work out how long each straight section will be.
 
Show your working here.

 
 
 
 
 
 
 
 
__________ m (to nearest metre)
Level:
5
Curriculum info: 
Maths, Geometry and Measurement, Measurement
Keywords: 
circles, rectangles, length, circumference, composite shapes
Description of task: 
Students calculate the lengths of circles and straight lines on an oval athletics track from its given radius and total length, and show their working.
Answers/responses: 
  Y10 (05/2007)
a)

Uses correct formula (πd or 2πr)
[Accept if answer is wrong]

219.8 (using π = 3.14)
[Accept 217-220]

very difficult

very difficult
b)

Correct method used i.e. [400 – answer to a)]/2

90 (using  π = 3.14)
[Accept 90-91.5 as it depends on what approximation of π is used]
[Accept if an incorrect answer to a) is used correctly.]

very difficult

very difficult
Based on a representative sample of 156 students.

NOTE: Accept answers, even if the student does not give answer to the correct number of decimal places.

Diagnostic and formative information: 
  Common error Likely misconception
a)
b)		 109.9 (or near this)
145 (or near this)		 Uses πr as the circumference of a full circle instead of πd  or Gives the length of just one of the two curves.
a) 3848.5 (or near this) Uses πr2 as the circumference of a circle.
a)
b)		 Various responses
Various responses		 Uses another incorrect method that incorporates π, often with computational errors
e.g., πr2 ÷ 2, π ÷ r, πr ÷ 2
a)
b)		 70 or 140
165		 Uses 2r (the diameter) as the formula for the circumference of a circle.
b) 180 Gives the length of both straights rather than just one.

NOTES:

  1. Several of these misconceptions are often combined, leading to a large number of different incorrect responses.
  2. Students find this question harder than when full circles are more clearly involved. This indicates that "composite lengths" is a more difficult problem for students. This is also true for composite areas and volumes.
Next steps: 
The first four diagnostics above or other incorrect answers indicate that the student does not know how to calculate the circumference of a circle.  These students need to have experiences in how to calculate the circumference. ARB resource Circumference and diameter and Figure it out resource Circle Links (Measurement, L4, Book 1, page 3) each give an activity that gets students physically measuring the circumferences and diameters of different circles and exploring the relationship between them.

The classical definition of π is geometrical, and it is the ratio between the circumference and the diameter of any circle.
π = circumference ÷ diameter          (of any circle)

So a circle with a diameter of 1 unit has a circumference of π units.

Students could also be asked to explore π (pi) on the internet. The following are some historical fractional approximations to pi:

Numerical value Fractional form Date and Origin
3 3/1 2000 BC Biblical
3.125 25/8 2000 BC Babylonian
3.16049 (16/9)2 2000 BC Egyptian
3.14285 22/7 250 BC Archimedes
3.14167 377/120 150 AD Ptolemy
3.14159292 355/113 480 AD Chung Chi

π =3. 1415926535897932384626433832795028841971….  (going on endlessly and "unpredictably")
In this resource we have used π = 3.14

Figure it out:
Circle Links, Measurement, L4, Book 1, page 3.