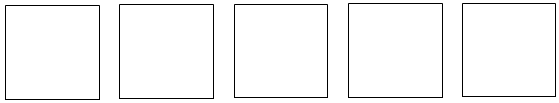
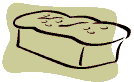
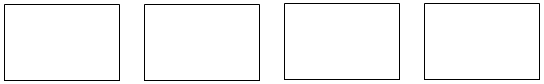
Partitioning pizza & fruit loaf

Partitioning pizza & fruit loaf

Pencil and paper
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about sharing food equally between people.

Some friends were sharing food at a party.

a)

Five square pizzas were shared equally between 6 friends.

i) Show how to work out how much pizza each person got.
Show your working (you may draw on the diagrams below).
 

 

 

ii) What fraction of pizza did each person get? _____
 
b)
Four fruit loaves were shared equally between 5 friends.

i) Show how to work out how much fruit loaf each person got.
Show your working (you may draw on the diagrams below).
 

 

 

ii) What fraction of fruit loaf did each person get? _____
   

 

Task administration: 
This task is completed with pencil and paper only.
 
Level:
4
Curriculum info: 
Maths, Number and Algebra, Number Strategies
Keywords: 
fractions, partitioning
Description of task: 
Students show or explain how to share equally amongst a number of people.
Curriculum Links: 
This resource can help to identify students' ability to apply additive or multiplicative strategies flexibly to whole numbers, ratios and equivalent fractions (including decimals and percentages).
Learning Progression Frameworks
This resource can provide evidence of learning associated with
Multiplicative thinking, sets 6-7
within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.
Answers/responses: 
  Y8 (05/2008)
a) i)

ii)

 Working e.g.,

or other working that demonstrates an understanding of
partitioning between 6.
5/6 (or 10/12 , 0.83, or 1/2 + 1/4 + 1/12 or 1/2 + 1/3 or 2/3 + 1/6)
[Also accept 1/6 as it is not incorrect, see note]		 easy

moderate
b) i)

ii)

 Working e.g.,

or other working that demonstrates an understanding of
partitioning between 5
4/5 or 8/10 (or 1/2 + 1/4 + 1/20 or 1/2 + 1/10 + 1/5)
[Also accept 1/5 as it is not incorrect, see note]		 moderate

moderate
Based on a representative sample of 219 students.
 
NOTE:

  1. Although 1/6 and 1/5 are correct for a) ii) and b) ii) respectively, this resource is about encouraging students to explore a single pizza/loaf as the referent whole resulting in a more useful and rich investigation into fractions as a division relationship.
  2. Students can also be asked about efficiency of cuts to minimise the number of cuts they need to do to share out the pizza/loaf.
Teaching and learning: 
Knowing that shapes and sets can be partitioned into equal parts is important for understanding the part-whole relationship between the numerator and denominator in fractions.  Also important is the idea of a fraction as a quotient (fractions as the result of a division, e.g., 4 shared between 5 is 4/5).  Read more information about the different "constructs" (or personalities) of fractions.

There are a number of different aspects to this assessment resource:

  • Partitioning evenly for a given number
  • Recognising and maintaining the referent whole (or unit)
  • Combining partitioned amounts (often uncommon denominator)

Classroom discussion
Students could discuss making the least number of cuts, and the referent whole that they have found the part for (is it one pizza/loaf? or is it all the pizza/loaf?).  To help students expand their own repertoire of strategies and develop their understanding about partitioning, have them explain, compare, and justify their strategies as a class or in groups. They could look at similarities and differences between the strategies and identify which are more sophisticated or efficient.  See Mathematical classroom discourse for more information on this assessment strategy.

Strategies
The most common strategy (40%) for partitioning the pizza/loaves was to partition each pizza/loaf into the parts required.  Students who use this method tended to indicate better mathematical ability than students who used other methods (other partitioning or indexing methods).  For example, students who began partitioning into halves or quarters were not necessarily choosing appropriate partitions.  Halving is the most basic partition and it is important that students explore other partitions that are more appropriate for the objects and the divisor, rather than select halves and quarters to divide an object.  This suggests that students should first focus on partitioning at their own level and then explore more appropriate efficient ways of partitioning the shapes (e.g., less cuts).

Diagnostic and formative information: 
Some students gave their answer as a number of fractions still to be combined.  As efficiency of cutting is sought this resource can provide a context for addition of fractions of unlike denominators (see Exploring more efficient partitioning below)
Next steps: 
Non-fractional answer
For students who had trouble naming the fractional part, more exploration with simple fractional partitions should help identify how to name or identify the part.  Partitioning resources at Level 2 or 3 may be useful for this.

Cannot add fractions with different denominators
For students who could not combine a number of fractions with different denominators, have them explore adding simple fractions. Click on the link for resources involving adding fractions or information about Adding fractions.

Using all the pizza/loaf as a referent whole
Over 10% of students answered 1/6 for question a) and 1/5 for question b).  This answer is actually correct if the referent whole is taken to be all the pizza/loaf.  However it is important to move students on to recognising there are 5 (or 4) pizzas (or loaves) and that shared amongst 6 (or 5) people is 5/6 (or 4/5).  This helps students develop an understanding that a fraction is also the result of a division (quotient).  For students who answer this way ask them what the whole was they have used and then ask them "How much of a pizza (loaf) does each person get?".  This is also true for students who answered 5/30 or 4/20 for a) and b) respectively.

Discuss how the answers, 1/6 and 5/6 (or 1/5 and 4/5), are different, and what makes them different.  Students should recognise that when the whole is 5 pizzas the solution is 1/6 and when the whole is 1 pizza the solution is 5/6 .  Students may also notice that the fraction is 5 times as big when the referent whole is one fifth the size.

Exploring more efficient partitioning
For students who could partition each cake and pizza into 3rds or 4ths and correctly identify what fraction of a cake/pizza each person got, ask them if there is a way to do fewer cuts when sharing the cake/pizza up.  They could start by exploring the least number of cuts and identifying the parts they have created, e.g., sharing 5 cakes between 6 people only involves 7 large cuts and 4 small cuts – indicating it is more efficient than cutting up each cake (25 cuts).

Then get students to work what parts they have: 1 whole, 1 half, and 1 quarter (and then how to add them).  This uses efficiency to drive different partitioning and requires students to add different fractions to work out the total share.  Click on the link for Adding fractions.

Other resources
For similar ARB resources, click on the link or use the keywords, fractions AND partitioning.
Click on the link for further information about partitioning and how it supports fractional understanding.