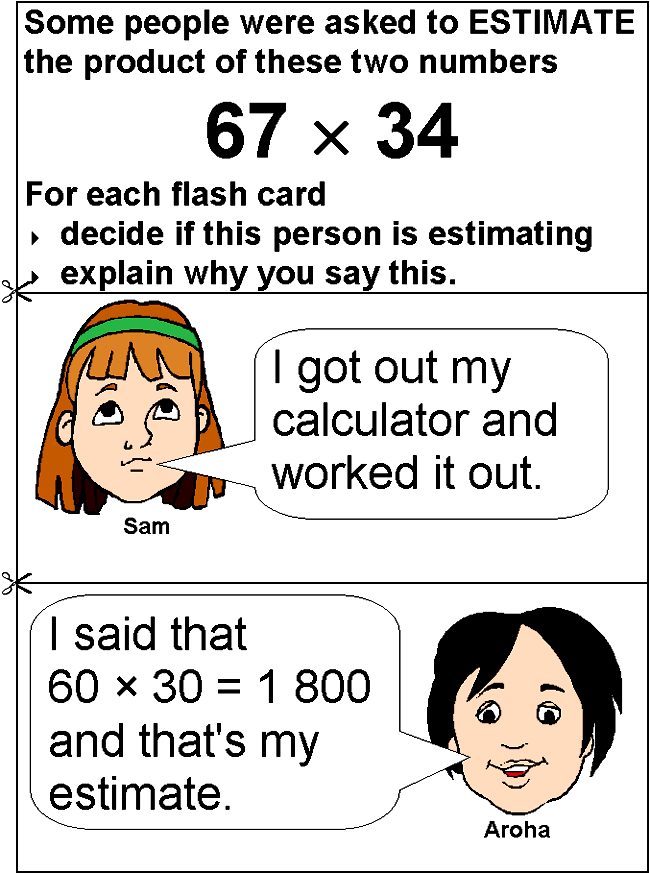
Who is estimating? Multiplication
Your teacher will show you responses from five people who were asked to estimate this multiplication:
67 × 34
Some of them were estimating and some were not. For each flash card:
- circle either the tick or the cross in the box to show whether that person is / is not estimating;
- explain your answer.
|
|||||||||||||||||||



 or
or  ) (Circle one)
) (Circle one)