Dividing by four and six
0
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about identifying numbers that are divisible by four or six.
Task administration:
This task can be completed with pencil and paper or online (with SOME auto marking displayed to students).
Level:
4
Curriculum info:
Key Competencies:
Keywords:
Description of task:
Students identify multiples of 4 and of 6, and explain their reasoning.
Learning Progression Frameworks
This resource can provide evidence of learning associated with within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.Answers/responses:
| Y8 | ||
| a) |
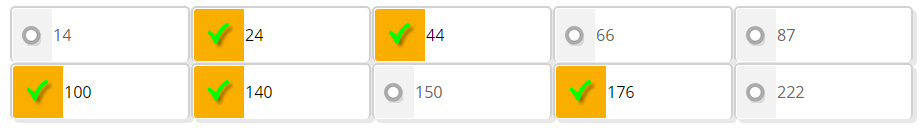
Explanation:
Explanation involving recognition of halving, and halving again (or an even number)
Examples
NOTE: checking each number by dividing through by 4 describes a "way to know" if a number is divisible by 4, but the intention of this task is to also move students to develop a more general conjecture or rule that can be easily checked.
|
difficult
(All 5 correct and none incorrect)
moderate
(4 correct)
|
| b) |
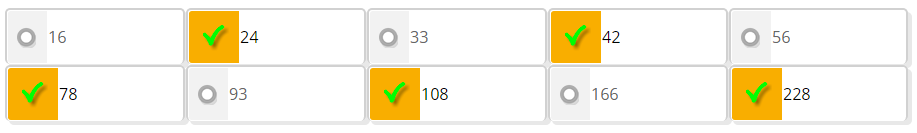
Explanation:
Explanation involving halving and then recognising divisibility by three
Examples
NOTE: checking each number by dividing through by 6 describes a "way to know" if a number is divisible by 6, but the intention of this task is to also move students to develop a more general conjecture or rule that can be easily checked.
|
difficult
(All 5 correct and none incorrect)
difficult
(4 correct)
|
| c) |
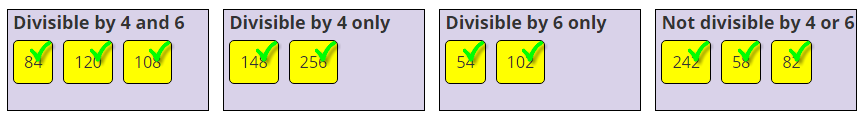 |
very difficult
(All 10 correct)
difficult
(more than 8 correct)
moderate
(more than 5 correct)
|
Diagnostic and formative information:
| Common error | Likely misconception |
|
a) not selecting 176
b) not selecting 78, 108 or 228
|
Student does not know how to divide by 4 or 6
Student does not know how to divide larger numbers by 4 or
does not have a rule they can use for divisibility by 4.
Student does not know how to divide larger numbers by 6 or does not have a rule they can use for divisibility by 6.
|
|
a) Selects 24 and 44
b) Selects 56 or 166
|
A number is divisible by a factor, if the last digit of the number is that factor
Students may think that if the last digit of a number is a 4 or 6, then the whole number is also divisible by 4 or 6.
|
| c) Puts 84, 108 or 120 into Divisible by 4 only or Divisible by 6 only - not both. |
Students only look for a single solution
Students stop once they have found that a number is divisible by 4 or 6. They do not check whether it is divisible by the other factor.
|
Question a)
Overall question a) was "difficult", as students were asked to identify all 5 numbers that were divisible by 4.
For question a) students found identifying 24 and 44 as divisible by 4 very easy, followed by 140, 19 (both easy), and 176 (moderate).
Sufficient explanations
Sufficient explanations involved recognition of halving, and halving again and/or refernce to even numbers.
Examples
- If the last two numbers are divisible by 4 then the whole number is divisible by 4
- Divide by two and if it ends in an even number it is divisible by four
- If it can be halved and then halved again it is divisible by 4
- If you can halve it twice, then it's divisible by four.
Partial explanations
Other explanations that showed some understanding, but described how students could calculate rather than a conjecture or general rule to know whether a number is divisible.
Examples
- I know my four times tables and that four can times into twenty so that helps you a bit
- Because it has to be even and I took time to divide each number by four
- Count by 4/counting up in fours;
- If the number is above 100, I already know that 100 fits into four, so i remove 100
- Because when you divide it by 4 there is no remainder.
Incorrect/incomplete explanations
- Because it has a four at the end
- It will have an even number (not a tens place)
Question b)
Overall question b) was also "difficult". Students were asked to identify all 5 numbers that were divisible by 6.
However, students found identifying 24 as divisible by 6 very easy, and 42 easy; followed by 108, 78, and 228 (all moderate). Divisibility by 6 is more complex than by 4, and can involve two steps (divisibility by 2 and then divisibility by 3; which could be halving and then adding all digits to find a factor of 3).
Sufficient explanations involved dividing by two (or halving) and then recognising divisibility by three.
Examples
- Halving and then being divisible by 3
- I check if it is divisible by 2 and then add all the digits together to see if it is divisible by 3
- If it is divisible by both 2 and 3.
Partial explanations
Other explanations that showed some understanding, but described how they calculated rather than a conjecture or general rule to know whether a number is divisible (e.g., counting, times tables or dividing each number to check whether it has a remainder).
Examples
- I took time to divide each number by six
- Counting in six
- Divide by 6
- Because when you divide it by 6 there is no remainder
- I know my six times table and knowing that 30 and 60 is in the six times table it helps.
- 4 × 6 = 24 and 6 × 7 = 42 [illustrates how each number is checked by multiplying by 6 and by the other factor to show divisibility].
Incorrect/incomplete explanations
- I do long division
- Skip counting
- I know my math.
Question c)
Overall question c) was "very difficult". Students were asked to correctly place all 10 numbers.
Students found correctly identifying 120 as divisible by both 4 and 6 easy. The number 256 was the most difficult number to correctly recognise for divisibility by 4 or 6. The numbers 84, 120, and 108 are divisible by both 4 and 6. They could have been harder to place as they could have been placed in either box if the students didn't check their divisibility by the boths factors. However these numbers were not notably harder to place than many others.
Next steps:
Student does not know how to divide by 4 or 6
Some students may still have difficulty working out division problems with larger numbers (in the hundreds). Students need to be encouraged to see the part-whole nature of numbers to help them identify the larger number factors of 4 (or 6). Students could then look at the larger numbers like 242, 256, 148 and break them down to numbers with more obvious parts (e.g., for divisibility of 4: 242 = 200 + 42; 256 = 240 + 16, etc; and for divisibility of 6: 108 = 120 - 12; 84 = 60 + 24). If each of these easier-to-work-with numbers is divisible by 4 (or 6) then under the distributive law (distributive law [Wikipedia]), so is the original number.
Students could also explore the resource Dividing by three and five to work with simpler factors.
A number is divisible by a factor, if the last digit of the number is that factor
Students who think that if the last digit of a number is a factor of 4 or 6, then the number is also divisible by 4 or 6 need to explore some counter examples.They could test if their rule works by dividing 2-digit numbers like, 14, 34, 26, and 46. Student also need to understand that a rule should work for all numbers.
Students only look for a single solution
Drawing attention to all the categories available in this task (divisible by 4 only, 6 only, 4 and 6, and neither) prior to occompleting it will help students to check the other categories before making a decision. For example, once students have noted that the numbers are divisible by 4, they can then be asked if 6 is also a factor (i.e., are they divisible by both 4 and 6?). NOTE: the category of Divisible by 4 and 6 has been put first to encourage the checking of both factors.
To support the idea that numbers can be divisible by a range of factors, students could be asked about simple numbers like 12 (2, 3, 4, and 6), and 36 (2, 3, 4, 6, and 9) and be asked to identify all the different factors.
They could also explore patterns of factors in Multiplication. See the Basic facts conceptual map to explore such patterns.
Partial explanations
Students who provided explanations that were incomplete can work with a peer to share and critique each other's explanation. Then co-construct an explanation and share back with the class. The goal would be to produce a sufficient explanation as a group or class. Having some guidelines about what constitutes a sufficient explanation might also support this, e.g., "the explanation needs to be sufficient, so that it helps someone work out divisibility wihtout having to divide through each number to check, but provides a faster way to know".
For the purposes of this assessment the explanation is not intended to be arduous. For example, sufficient explanations for knowing a number is divisible by 4 are:
- If the last two numbers are divisible by 4 then the whole number is divisible by 4
- Divide by two and if it ends in an even number it is divisible by four
- If it can be halved and then halved again it is divisible by 4
- If you can halve it twice, then it's divisible by four.
These all provided a rule that makes checking divisibility a matter of quick mental arithmetic - rather than having to individually calculate each number by 4.
In addition a sufficient explanation might involve noting that because 100 is divisible by 4, only the last two digits (tens and ones) of any number need to be considered to know whether the entire number is divisible by 4.
Providing an explanation about being divisible by 6 was more complex, as it involved two steps: recognising the prime factors of 2, and then 3.
Examples:
- Halving and then being divisible by 3
- I check if it is divisible by 2 and then add all the digits together to see if it is divisible by 3
- If it is divisible by both 2 and 3.
These all provided a rule that made checking easier, but relied upon recognition of divisibility by 3.
Divisibility rule for 3
There are divisibility rules for 3 (https://en.wikipedia.org/wiki/Divisibility_rule#Divisibility_by_3_or_9), but students should be given the chance to work out their own strategies for solving divisibility before this is explored. Students could then explore why this rule works. Students could try a range of numbers that are or are not divisible by three. They could then make conjectures about what makes a number divisible by 3 (e.g., all the digits are divisible by 3). They could then test their conjectures on other numbers.
For some background information around divisibility by 3, see the Khan Academy:
For further information about divisibility tests, refer to the following:
- Divisibility test, Book 8: Teaching Number Sense and Algebraic Thinking)
- http://en.wikipedia.org/wiki/Divisibility_rule
See the Basic facts conceptual map to explore the patterns for factors in Multiplication.
Divisibility rules for 3 and 9 on Wikipedia
For some background information around divisibility by 3, see the Khan Academy:

