Sharing counters

- This resource is designed for small group work, where the teacher can observe how students set up and partition the sets.
- How students partition is an important part of this resource. It can identify students that are using counting strategies to solve sharing problems.
- Make a note of students who set up the counters as arrays, single lines or clusters.
- Ask students if they can identify other equal-sized groups they could put the counters into.
NOTE: The questions ask students to partition a set into a number of groups. Dividing counters into groups of a given size and asking how many groups is a different type of division, called quotition. For example "How many groups with 2 counters in them could you make with the counters?"
| a) |
i) ii) |
4 2 |
|
| b) |
i) ii) |
6 3 |
|
| c) |
i) ii) |
5 3 |
|
| d) | 1/4 | ||
NOTE: Encourage students to use the word fraction rather than part, as future fraction problems may ask for top heavy (or improper) fractions, e.g., 5 quarters, which is not so intuitively a "part".
For students who cannot use a strategy to share the counters equally, introduce the idea of sharing amongst people and what would be fair. Ask “Is someone getting more than someone else?”. Explore this equal sharing with shapes and sets of countable items. Encourage students to think about how to lay them out to make breaking them into groups easier. Start with small numbers divided into small groups, e.g., 6 counters into 2 groups, and then ask them to explain how they worked it out. Then suggest they use this same strategy for larger numbers of counters and groups.
Using a counting strategy
Students who line the counters up and count out the counters into the groups then count the number in each group are using a counting strategy, e.g.,
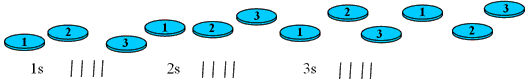
This indicates that they understand the concept of equal-size and equal sharing (see resource Equal sharing with countable items).
Once they can use this strategy successfully, encourage students to think about other strategies to share the set of objects between the groups without counting. For example, using an array may help students visualise the groups that make up a set, or asking children what they know about the number of counters in the set and number of groups required, e.g., "If there are 12 counters and 3 equal-sized groups, what do we know about breaking 12 into 3 parts?"
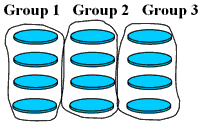
NOTE: After students can arrange counters to solve equal sharing problems, encourage them to visualise the counters in their head without needing the counters (called imaging).
Sharing equally
For students who can share the set into n parts and identify the division relationship of partitioning, ask them to name what fraction one part of the set is, e.g., "what fraction of the whole set is one of the groups?"
Students may know that 12 into 3 groups is
12 ÷ 3 = 4
which means there is 4 in each group
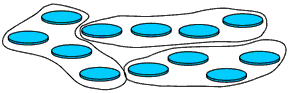
This encourages students to recognise that a fraction is a part of a whole (part whole understanding of fractions). This whole can be a set, a region or a number.
Equal sharing of sets, numbers or regions for simple problems and using a matching strategy is at Stage 4: Advanced counting - Stage 5: early additive part-whole. Students who can construct and name non-unit fractions are at Stage 5: early additive part-whole (using repeating addition) - Stage 6: Advanced additive (partitioning using division) part-whole.

