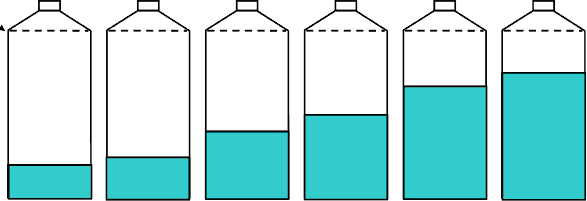
Ordering filled bottles

Ordering filled bottles

Auto-markingPencil and paperOnline interactive
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about knowing how large fractions are.
These bottles are in order from least full to most full. 
bottles-different-filled.png

Question Change answer

Put the correct fraction under each bottle to show what fraction it is full. 
Image counting main image
Task administration: 
This task can be completed with pencil and paper or online WITH automarking.
Levels:
3, 4
Curriculum info: 
Maths, Number and Algebra, Number Knowledge
Keywords: 
fractions, ordering fractions
Description of task: 
Students match given fractions to images of milk bottles to show how full each bottle is.
Answers/responses: 
    Y6 (11/2006)
ordering-filled-bottles-answers.png
[an error could be a transposition, swapping, or scribing error]
 For all 6 correct

For 1 error

 moderate

easy
Teaching and learning: 
This resource uses representations to support student visualisation of fractions.  It is intended to support their understanding that fractions have a size and can be compared.  Milk bottles and their fullness is a common context for many children.  It may help them make connections between fractions and their informal language of proportion.
Diagnostic and formative information: 

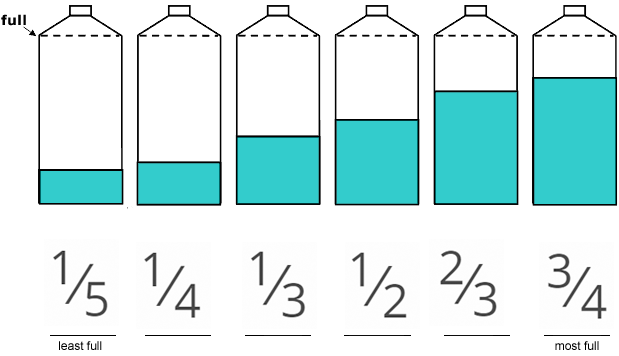
In order to develop an understanding of fractions, it is important that students experience different types of fractions – not just unit fractions, but non-unit fractions up to 1 (i.e., proper fractions).  Being able to only order unit fractions may result in students developing and consolidating related misconceptions about fractions.

Half
About four-fifths of students correctly labeled the fourth bottle as 1/2 . This may indicate their prior knowledge about the size of a half.  Conversely, a very small number placed the 1/2 as the first fraction indicating their lack of understanding about the quantity of a half (the most common fraction).

Three quarters
Nearly two-thirds of the students placed 3/4 in the correct place (the last bottle).  This could be because they were aware that 3/4 is greater than 2/3 .  However, students could have selected the right answer for the wrong reason: 3 is the largest numerator so it must be the largest, or 3 and 4 can be added or multiplied to be larger than any other numbers.  It is important to ask students to explain why they know where the fraction goes and how they know that one is larger or smaller than adjacent fractions.  The reasoning and strategies used can provide valuable insight into their fractional understanding.

One fifth
Over two-thirds of students correctly labeled the first bottle as 1/5 .  This is likely to indicate they understand that 1/5 is the smallest fraction, and have some exposure to rules for unit fractions like the bigger the number on the bottom the smaller the fraction – this is still not a sufficient indication of really understanding why 1/5 is the smallest and students should be asked to explain their reasoning.

Common error Likely misconception
1/2 , 1/4 , 1/5 , 2/3 , 2/5 , 3/4
or
1/2 , 1/4 , 1/5 , 2/3 , 3/4, 2/5		 Whole number misconception
This misconception is about the relationship between the denominator and numerator. Students may try to relate the two numbers by ordering the numerators and then the denominators either in descending or ascending order.

Students believe that the smaller the numbers in the fraction the smaller the fraction. This "rule" works for whole numbers, but does not apply to all rational numbers.
NOTE: all the unit fractions are less than all the non-unit fractions.
1/5 , 1/4 , ... A derivation of above. Students are likely to have a whole number misconception about fractions, i.e., that 5 is greater than 4 so 1/5 is greater than 1/4 , but adapt it to place the fractions they know.
..., 2/5 , 1/4 , ... Non-unit fractions misconceptions
Students correctly order all unit fractions, but swap 2/5 and 1/4 . Students may simply have no strategy to compare 2/5 and 1/4 , and revert to a whole number misconception where, because the numbers 2 and 5 are greater than 1 and 4, then this means that 2/5 is also greater than 1/4 . 
NOTE: About one-fifth of students indicated that 2/5 was greater than 1/2 . This would indicate that because the numbers 2 and 5 are greater than 1 and 2 then this means that 2/5 is also greater than 1/2 .
..., 3/4 , 2/3 , ... Students may correctly order all unit fractions, but swap 3/4 and 2/3 . This swap is likely to be that students have some understanding about the larger denominator meaning smaller pieces, but have not developed an understanding of fractions as part-whole relationships, and therefore cannot correctly order non-unit fractions.

NOTE:

  1. Students may make some of these errors in combination with each other.
  2. Some students may not actually consider the other fractions in the placement.  They may simply match the fraction to the shaded bottle without thinking about the order of the fractions. …
Next steps: 
Students who have the whole number misconception identified above need to develop a part-whole understanding of fractions before trying to devise a system to order or compare fractions.  A part-whole understanding of fractions is about recognising that a fraction is a part of a whole, and that it is the relationship between the top and bottom number that describes and quantifies the fraction, i.e.,  it is about the relationship between the numbers, not their absolute size.  Students should be aware that the bottom number represents how many parts make up the whole, and the top number represents how many of these parts are of interest. For more information on this , click on Fractional reasoning concept map, and go to the section Fractions as part-whole relationships.

If required, students could go back to partitioning and explore constructing the parts (unit fractions), combining these parts to make non-unit fractions, and naming these new fractions.  Encourage students to explore a range of many new fractions such as 1/8 , 3/7 , etc.  After this, encourage students to compare just two fractions (including non-unit fractions) before trying to order a number of unit and non-unit fractions.  Appropriate drawing of fractions can promote understanding and help with comparing the size of fractions. For more information on this , click on Fractional reasoning concept map, and go to the section Partitioning and divided quantities.

For students who misplace one fraction such as 2/3 , 3/4 , or 1/5 , ask them to explain how they know that the misplaced fraction is larger or smaller than adjacent fractions.  For a resource comparing fractions see NM0138 Larger fractions.

For students who could place all six fractions correctly, ask them where they think 3/5 , 4/5 , or 3/2 may fit in the order.  Ask students to explain their reasoning.

NOTE: Students may also be having difficulty with the fraction notation and it may be prudent to be aware that their prior experience may involve the name but not the notation.  For example they may know how much three-quarters is, but not that 3/4 is three-quarters.

For more information about fractions as part-whole relationships click on the link Fractional concept map, and go to that section..