Ordering improper and mixed fractions
0
Overview
Using this Resource
Connecting to the Curriculum
Marking Student Responses
Working with Students
Further Resources
This task is about ordering fractions correctly.
Task administration:
This task is completed online and has some auto-marking displayed to students.
Copyright:
Graphics copyright (c) NZCER
Level:
4
Curriculum info:
Keywords:
Description of task:
Students identify the larger of two improper fractions and drag a number of improper and mixed fractions into ascending order.
Curriculum Links:
This resource can help to identify students' ability to apply multiplicative strategies flexibly to fractions.
Learning Progression Frameworks
This resource can provide evidence of learning associated with within the Mathematics Learning Progressions Frameworks.
Read more about the Learning Progressions Frameworks.Answers/responses:
| Y8 (11/2015) | ||
| a) |
11/3 is larger than 11/4
Explanations:
Students used a range of sufficient explanations that involved utilising equivalence, converting to mixed fractions to make it more plain, discussing the size of the parts, and some logical discussion, e.g.,
|
very easy |
| b) |
5/4 is larger than 9/8
Explanations:
Students used a range of sufficient explanations that involved utilising equivalence, converting to mixed numbers to make it more plain, discussing the size of the parts, and some logical discussion, e.g.,
|
easy |
| c) |
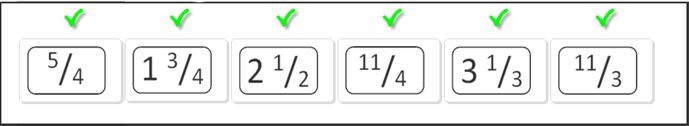 |
moderate
|
Teaching and learning:
This task involves comparing and then the ordering of improper and mixed fractions. Underlying this is the idea of fractions as numbers (rational numbers) that can be compared and put onto a number line. Ordering fractions normally resides in Number knowledge strategy, but this task requires students to use multiplicative strategies to work out the relationships between the numerator and denominators.
For more information about the different representations of fractions see the Fractional Thinking Concept Map.
Diagnostic and formative information:
Common errors
Question a)
Some students identified that 11/4 is larger than 11/3 with a range of explanations:
- bigger number in the fraction means it is a bigger fraction (because 4 is bigger than 3),
- it is about the number of pieces you get, so you get more pieces, which makes it bigger
- some misconceptions around elevenths (i.e., or because there are more elevenths, 11/4 means more elevenths, which is more).
Question b)
- Some students identified that 9/8 is larger than 5/4 with explanations that involved the numerical value of the digits was greater, more pieces meant the fraction 9/8 was larger, or they incorrectly used a cross multiplying strategy to get a common denominator strategy.
- Some identified that they were the same size based on the fact that they were one piece more than a whole without considering the size of the piece.
Common explanations to justify how students knew one fraction was larger than another.
Students used a range of sufficient and incomplete explanations that involved utilising equivalence, converting to mixed numbers to make it more plain, discussing the size of the parts, and some discussion about the part-whole nature of fractions. Some examples of the explanations across questions a) and b) are shown below
Examples of “sufficient” explanations
Converting to mixed fraction/percentage
Question a)
- 11 thirds is 3 and 2 thirds and 11 quarters is 2 and 3 quarters
- 11/3 is bigger because 11 divided by three is 3.67. 11 divided by four is 2.75.
- Because 11/3 converted to a mixed fraction is 3 2/3 and 11/4 converted is 2 3/4 so 11/3 is larger
Question b)
- 5/4 is bigger because converting these into percentages proves it
- Cause 4 divide by 5 is 1 1/5 that is bigger than 9/8
- 5/4 makes 1 and 1/4 while 9/8 makes 1 and 1/8 . Since 5/4 has a larger quantity, it is bigger than 9/8 (i.e. 1/4 is greate than 1/8)
Utilising equivalence
Question b)
- Because I converted both of the fractions into eighths and 5/4 was the bigger fraction
- Double 5/4 then it turns into 10/8 , so 5/4 is bigger than 9/8
Working with the size of the parts
Question a)
- Top numbers are the same so that means that if one bottom number is the smaller it [the fraction] is bigger
- The two numerators are the same, whilst the two denominators aren't. 3 is smaller making the pieces bigger.
Question b)
- Same amount of wholes but the 5/4 fraction has a bigger pieces
Discussion about the part-whole nature of fractions
Question b)
- Because if the numerator is one number higher than the denominator, the fraction with the smaller denominator is larger
- Imagine four pizzas if two pizzas were cut into quarters and I ate five quarters there would be 3/4 left, whereas 9/8 there would be 7/8 left so 5/4 is bigger.
- 9/8 is 1/8 away from a whole, but 5/4 is 3/4 away from 2 wholes.
Examples of incomplete/developing explanations
While students may have given the correct answer, their explanations were not always complete or “sufficient”.
Question a)
- 11 Thirds is bigger as thirds are bigger than quarters - missing the point that "the numerator is the same therefore ..."
- 1/3 is bigger than 1/4
- 11/3 greater - The denominator is smaller which means we have more wholes.
- because the denominator is smaller making the piece bigger
- because 11/4 has smaller numbers when you convert it into percent
- because if you reduce them to mixed fractions, 11/3 is larger
- the denominator is smaller in which case it makes the amount bigger
- if you convert both fractions, 11/3 has a bigger amount than 11/4
- 3x4=12 so 11/4 is two wholes and 3 quarters
Question b)
- 1/4 is bigger than 1/8
- 5/4 has 4 while 9/8 has 8.
Examples of explanations with incorrect components
Equivalence
Question b)
- Because if you find an equivalent fraction and you times the denominator by the same number you find that 9 eighths is bigger
Incorrect understanding of part-whole nature of fractions
Question a)
- If a pizza was cut into 11 pieces one of the options you could get 4 pieces of the pizza and one piece you would get three so therefore 11/4 is bigger
- 11/3 is greater - I plast 1 to the 11 and the 3 in 11/3 to see what one is bigger
- [11/4 is greater than 11/3] because 11 fours are is 1 eleventh bigger than 11 threes
- takes up less to make a third
Question b)
- 9/8 is larger because 9/8 is smaller peaces than 5/4 but there are more peaces.
- If it was a pizza 9/8 just has more pieces.
- Because there are less parts in 1 quarter is less than a eighth
- Size of the pieces is not worked out correctly
- They are both the same because they are both 1 whole and 1 piece
- They both need 1 more to make a whole
- I think they're both the same because I think 4/8 are the same as 1/4 .
Next steps:
Treating fractions as natural numbers
Students identified who identified that 11/4 is larger than 11/3 (or 9/8 is larger than 5/4) and explained that the "bigger number in the fraction means it is a bigger fraction" are applying the rules for the natural number system to fractions. Student could explore what the numerator and denominator mean in a fractional (Rational number) sense by looking at partitioning and constructing the fraction name. Similarly, students who identified that there were more pieces, which meant the fraction would be larger, could be asked to explore examples of partitioning. The level 2 resources Dividing fractions, Dividing squares and Cutting the cake all explore partitioning into different numbers and identifiying the fraction created. Students could then be asked to develop a conjecture of what it means when the denominator is larger and what happens to the size of the fraction. Ultimately, they need to understand that if there are more pieces of a whole, then the pieces (how much each person gets) will be smaller, and, furthermore, that these relationships can be shown using a fraction number.
The resources Fractions on a number line and Fractions on number lines could be used to develop a sense of the size of fractions by ordering them on a number line.
Identifying the correct fractions
Some students interpeted the fractions 11/4 and 11/3 as elevenths and some argued that because there were more elevenths 11/4 was greater. This may be a simple mistake or an indication of a deeper misunderstanding about the part-whole nature of fractions as numbers. Students could be asked to write some common fractions to ensure they know the convention of fractional notation. Starting with simple fractions and then moving into 4 fifteenths, etc, to show the patterns of a [count of parts]/[number of parts in the whole]. In addition another way to to consider exploring the meaning of fractions is 5/7 as 5 lots of 1/7 . This way of constructing more complex fractions by counting or multiplication can support students understanding about the size of a fraction as a number.
Insufficent explanation
Students who could not provide a sufficient explanation of why one fraction was larger than another could be encouraged to compare two simpler fractions and draw pictures/diagrams to show their thinking, and then explain to a peer. They could also work in a small group to develop an explanation back to the class. Having explicit guidelines for what a sufficient explanation might contain would support them. Students could develop their explanation using examples. The assessment resources Larger fractions, Larger fractions II, Larger fractions III ask students to identify which is larger and why.
Click on the link for more information and resources in the Fractional Thinking Concept Map

